One Thousand is Unfair, Two Thousand is Fair

The Olympic 500-m and 1000-m sprint competitions are the Formula One events of speedskating, watched by millions on television along with the fans in the stands. The athletes skate in pairs, in inner and outer lanes, switching lanes on the exchange part of the track; see the figure below. They reach phenomenal speeds of up to 60 km/h and naturally experience difficulties negotiating the curves, which have radii of ca. 25 m and ca. 29 m. Feel free to try it yourself, at the nearest Olympic rink; at any rate you may start by putting in your own weight $m$ and then compute the centripetal force of acceleration $mv^2/r$ with speed $v=15\,{m/s}$ (15 metres! per second!), during the outer turn (ouch) and then during the inner turn (double-ouch).

The 500-m race: last inner vs. last outer

For the 500-m (one lap + 100 m), which takes a top male athlete less than 34.5 seconds and the best female skaters less than 37.0 seconds, the last inner turn is typically the most difficult part of the race, as this book excerpt (from Bjørnsen, 1963, p. 115, and in my translation) testifies:

He drew lane with anxious attentiveness – and could not conceal his disappointment: First outer lane! He threw his blue cap on the ice with a resigned movement, but quickly contained himself and picked it up again. With a start in inner lane he could have set a world record, perhaps be the first man in the world under 40 seconds. Now the record was hanging by a thin thread – possibly the gold medal too. At any rate he couldn’t tolerate any further mishaps.

^{Евгений Гришин, four-time Olympic winner, and world record holder for the 1000 m from 1955 to 1966. One of his other books has the Kierkegaard title "Ili-ili".}

The problem, for Yevgeny Grishin and the other sprinters, is that of the centrifugal forces (cf. the acceleration formula $a = mv^2/r$) and meeting such challenges precisely when fatigue starts to kick in, making skaters unable to follow the designated curve and in effect skating a few metres extra. The Grishin excerpt also points to the fact that luck can have something to do with one’s result; for the 500 m many skaters prefer the last outer lane (that is, starting in the inner) to the last inner lane (starting in the outer). Thus the Olympic 500-m event has carried a certain random element of potential unfairness to it, as a random draw determined whether a skater should have last inner or last outer lane. Via valiant statistical efforts I was then able to demonstrate, in a 1994 report, that the difference between last inner and last outer is statistically and Olympically significant, to the tune of ca. 0.06 seconds (enough of a difference to make medals change necks). Owing in part to these efforts and detailed reporting, along with careful deliberation and negotiations within the International Skating Union (ISU) and the International Olympic Committee (IOC), these Olympic all-on-one-card rules were finally changed. From 1998 Nagano onwards, the skaters have needed to race the 500-m twice, with one start in inner and one in outer lane, and with the sum of their times determining medals and the final ranking.

The 1000-m race: 3 inners + 2 outers vs. 2 inners + 3 outers

This blog post is about the 1000-m, however. The two-and-a-half-lap race looks more asymmetric than the 500-m. If you start in inner, you'll have in in out out in before you cross the finishing line, whereas your compatriot in the race will have out out in in out. The speed is even higher than in a 500-m race; 34.00 might give you Olympic gold, but ten skaters might be better than 1:08.00. This may sound odd, but is due to the flying start effect; top skaters need a bit of time and a good stretch in front of them to get to maximum speed (and you don't need to start all over again after 505 metres of your 1000-m race). Thus the inner guy has perhaps a slight advantage in the start, with a longer straight stretch before hitting the curve. We'll soon enough look at data and models and the (tiny) differences between inners and outers, but another inner-factor that favours some skaters (though not all) is that when fatigue sets in and all your muscles shriek for an easier ride through the troubled aching turn, then inner might be better for you.

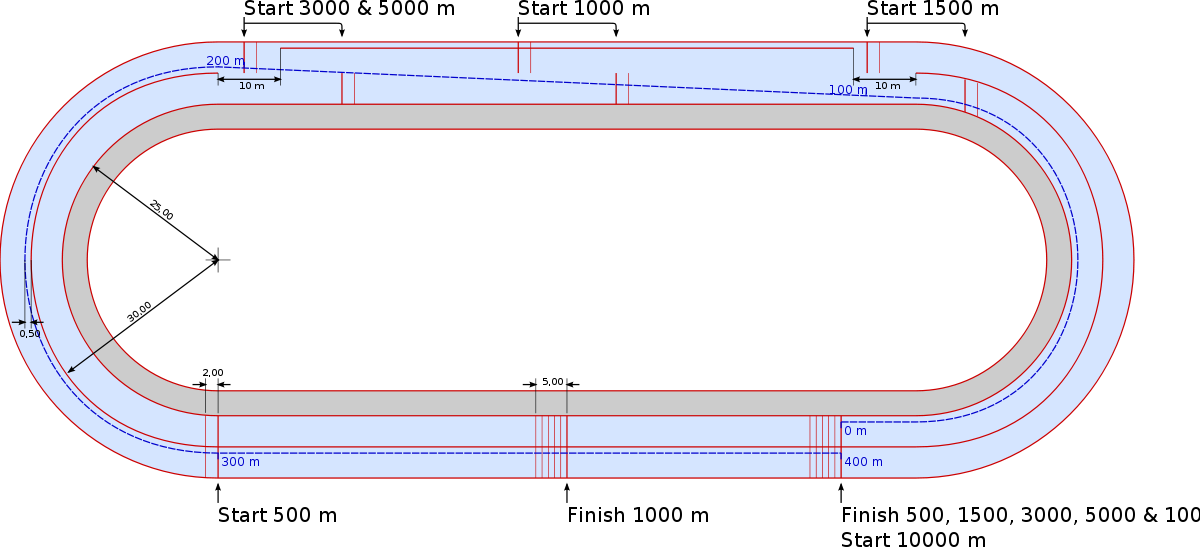
^{Higher geometry: Think through your two-and-a-half-lap race, if you start in inner lane (then ending in inner lane too), or in outer lane.}

So, though an Olympic or World Championship 1k certainly can be won by a top skater starting in the outer lane, many prefer and hope for being blessed by starting in the inner lane. That's the Burning Olympic Flame Question I'll address below, whether there is a perhaps slight but statistically and Olympically difference at work. In that case (well, surprise!, I plan to convince you, below, that there is such a difference), it should not and cannot be expected to work in the same way, for all skaters, from occasion to occasion. Rather, I'm after a statistical average-across-skaters parameter, say

\(d=\hbox{average time lost by being outer guy compared to if you could have been inner guy},\)

which by its nature is small, i.e. close to zero (if it had not been small, it would have been detected and agreed upon some 30 years ago). To make such a $d$ parameter well defined, and identifiable and estimable from data, I need relevant good-quality data, along with a proper statistical model. Again, the $d$ looks a bit counterfactual, and can't easily be assessed from results for one given race; also, its value (when I find it, below) doesn't apply to all skaters; rather, it's an average-across-top-skaters value (in well executed races without falls or mishaps).

In order to grasp the scale of things, where skaters run 15 metres per second and a full kilometre in 67 seconds, checking a few result lists shows that medals can easily change necks with the tiniest of time adjustments. This list is from the Saturday 1k race of the World Sprint Championships in Calgary 2017 (a dataset also returned to below), with the first four having started in the inner, the two next in the outer. So if there's a tiny inner advantage, the outer guys would take notice.

\(\eqalign{ \qquad 1\ \ &{\rm i} &{\rm K\ Nuis} &1.06.61 \cr \qquad 2\ \ &{\rm i} &{\rm V\ de Haitre} &1.06.72 \cr \qquad 3\ \ &{\rm i} &{\rm K\ Verbij} &1.06.73 \cr \qquad 4\ \ &{\rm i} &{\rm HH\ Lorentzen} &1.07.08 \cr \qquad 5\ \ &{\rm o} &{\rm N\ Ihle} &1.07.16 \cr \qquad 6\ \ &{\rm o} &{\rm J\ Garcia} &1.07.83 \cr}\)

The Annual World Sprint Bigaußian Fixed and Random Effects Championships

The annual World Sprint Championships provide the perfect type of data I need for comparing inners with outers and for setting up good models for identifying, estimating and assessing the $d$ parameter. In these two-day races (500 + 1000 on the Saturday, and 500 + 1000 on the Sunday), a wise ISU rule sees to it that each skater switches start-lane, from the first to the second day. Hence the races are symmetric and fair; the best skaters need to be prepared for both types of races; and no skater can complain of bad luck by the draw. Thus Håvard Holmefjord Lorentzen had i for inner on the 1st day and o for outer on the 2nd day, in the Calgary 2017 World Sprints (where he for two brief minutes held the world record in the four-distances sprint combination, the first time a Norwegian had managed such a feat, since 1973). We also have passing time (and hence lap time) data, for each skater, as indicated below (these are the top 6 of 25 skaters with valid results on both 1000 metres).

\(\eqalign{ \def\ii{{\rm i}} \def\oo{{\rm o}} \qquad & &{\it Saturday} & & & &{\it Sunday} \cr \qquad 1 &{\rm K\ Verbij} \quad &\ii &16.35 &40.96 &66.73\quad &\oo &16.50 &41.29 &67.94 \cr \qquad 2 &{\rm H\ Lorentzen} \quad &\ii &16.62 &41.39 &67.08\quad &\oo &16.50 &41.32 &67.38 \cr \qquad 3 &{\rm K\ Nuis} \quad &\ii &16.65 &41.09 &66.61\quad &\oo &16.63 &41.13 &66.51 \cr \qquad 4 &{\rm R\ Mulder} \quad &\oo &16.37 &41.04 &68.07\quad &\ii &16.40 &41.39 &68.23 \cr \qquad 5 &{\rm M\ Poutala} \quad &\ii &16.24 &41.26 &67.96\quad &\oo &16.38 &41.17 &67.97 \cr \qquad 6 &{\rm L\ Dubreuil} \quad &\oo &16.25 &40.98 &68.07\quad &\ii &16.34 &41.17 &67.77 \cr}\)

The are several statistical venues now, for coming at the $d$ parameter, the simplest of which might be to compare the 25 results with inner (mean 1:08.27) with the 25 results with outer (mean 1:08.51); yes, there appears to be a 0.24 sec advantage for inner, but the standard error we need to divide with, to carry out a simple t-test, is 0.26, so the t ratio is merely 0.91, and not significant. A more informative analysis is by regressing the result differences, say $y_2-y_1$ for Sunday minus Saturday, on the inner-outer covariate, and perhaps a few more. This is proving more valuable, and gives a clear, significant $d$ in favour of the inner. 

We may do better, however, by building a coherent statistical model for the $(y_1,y_2)$, incorporating all relevant information, from 200- and 600-passing times to the variability structure (which should encompass both day-to-day variation and skater-to-skater variation), and, crucially, the inner-outer-information. My considered model takes this form, for 1st race and 2nd race results $(y_{i,1},y_{i,2})$ for skater no. $i$:

\(\eqalign{ y_{i,1}&=a_1 + bu_{i,1}+cv_{i,1}+\hbox{$1\over2$}dz_{i,1} +\delta_i+\varepsilon_{i,1}, \cr y_{i,2}&=a_2 + bu_{i,2}+cv_{i,2}+\hbox{$1\over2$}dz_{i,2} +\delta_i+\varepsilon_{i,2}, \cr}\)

with $(u_{i,1},u_{i,2})$ passing times after 200 m, $(v_{i,1},v_{i,2})$ passing times after 600 m; $\delta_i$ a parameter following skater $i$, modelled as from a ${\rm N}(0,\kappa^2)$ distribution, and $(\varepsilon_{i,1},\varepsilon_{i,2})$ are independent error terms, from a ${\rm N}(0,\sigma^2)$ distribution. Crucially, the inner-outer information is taken care of via $(z_{i,1},z_{i,2})$, with $z=-1$ if start in inner and $z=1$ if start in outer. If $d=0.12$, for example, it means a time adjustment of $-0.06$ seconds for the inner guy (good) but $+0.06$ for the outer guy (bad).

The race-to-race variation is in the $\varepsilon_{i,j}$, identified with the $\sigma$, whereas the skater-to-skater variation is in the $\delta_i$, identified with the $\kappa$. A splendid skater has a negative $\delta_i$ (and a not-so-splendid skater has a positive $\delta_i$), though we do not attempt to estimate it directly, for each skater, only through the variation among skaters. I have also slightly modified the variables here, to subtract overall means of $u_{i,1}$ and $u_{i,2}$ in their definitions, and similarly for the $v_{i,1}$ and $v_{i,2}$; this eases interpretation and also helps stabilise numerics. In particular, $a_1$ and $a_2$ may now be seen as the overall expected levels on the 1st and 2nd day of the competitions. Parameter $b,c,d$ are regression structure parameters, signalling the effect of $u$ and $v$ and $z$ on the overall results, whereas $\kappa$ and $\sigma$ relate to variability and inter-skater correlation. 

The model has both fixed effects, related to the $a_1, a_2, b, c, d$ parameters, and random effects, via the skater-parameter $\delta_i$. I use $a_1$ for race 1 and $a_2$ for race 2, since racing conditions might differ, in terms of temperature, humidity, etc. (and wind, if outdoor). The model thus have seven parameters, and may also be represented as

\(y_i=\pmatrix{y_{i,1} \cr y_{i,2} \cr} \sim{{\rm N}}_2(X_i\pmatrix{a_1 \cr a_2 \cr b \cr c \cr d \cr}, \pmatrix{\sigma^2+\kappa^2 & \kappa^2 \cr \kappa^2 &\sigma^2+\kappa^2 \cr}), \)

for skaters $i=1,\ldots,n$, with the appropriate $2\times5$ covariate matrix $X_i$. Given the combined considerable efforts of the 25 skaters sprinting away in Calgary, World Sprint 2017, I can now fit and assess the model, and, in particular learn whether the world was unfair then, by seeing if $d$ was close to zero or not. 

Estimating and assessing the Olympic Unfairness Parameter

I do not go into the technical details here, but given the data and the model I've built above, I can use statistical methodology to

• (i) fit the model, i.e. estimate regression parameters $a_1,a_2,b,c,d$ and variance parameters $\sigma,\kappa$;
• (ii) assess the relevant uncertainty, in particular setting up standard errors and say 95% confidence intervals;
• (iii) screen out any outliers (and I do not wish an accidental mishap for one or two of the skaters to mess up the analysis and the conclusions):
• (iv) assess the full fit of the model.

Formulae and derivations and details and relevant discussion can be found in the Schweder and Hjort CLP book (2016, Ch 14). The basic estimation method is that of maximum likelihood, along with formulae for variance matrices, etc. Also, various model adequacy checks pan out very well (after careful screening away of outliers).

Note here that even though we might only care for $d$, the unfairness parameter, it is part and bargain of the statistical modelling and methods that I somehow need to factor in the other six parameters too (there's no free cake way of finding $d$ directly). For Calgary World Sprint 2017 I find $\widehat d=0.282$ seconds, a rather drastic estimate for the unfairness parameter, with estimated standard deviation 0.102. This translates to a t ratio of 2.776, a p-value for testing fairness ($d=0$) of 0.003, and a 95% confidence interval of $[0.083,0.481]$.

This is already pretty convincing, but cannot be taken at statistical trust value just yet, since this estimate, though significant and worrisomely big, has been obtained for just one World Sprint, that of 2017; this is also reflected in a rather wide confidence interval. The standard errors go down like $1/\sqrt{n}$, with $n$ the number of skaters, so we need more volunteers, among the top sprint skaters of the world, to give us more lovely races and hence increased statistical precision. The analysis is strengthened, considerably, by going through several World Sprint events. I have done so, for a list of such events, and will here briefly summarise results for the ten last World Sprint Championships, from 2017 backwards in time to 2008.

Displayed in this figure are point estimates $\widehat d_j$ (red dots), event by event, for the underlying unfairness parameters $d_j$, from Heerenveen 2008 to Calgary 2017. I also give 95% confidence intervals for each event, based on the statistical machineries of models, probability calculations, and data. The overall picture is pretty convincing; fairness would mean that the zero line $d=0$ should be covered by these intervals, for most of the events, and clearly it is not.

A fuller analysis is pointed to below, with a configram constructed for ten individual confidence curves, one such ${\rm cc}(d_j)$ for each of the World Sprint events, along with a bigger and fatter overall confidence curve, say ${\rm cc}^*(d_0)$, for the overall unfairness parameter $d_0$. This is the more narrow confidence curve in the middle, pointing to the grand overall estimate $\widehat d_0=0.187$ seconds. A 99% confidence interval for this overall $d_0$ is found to be $[0.108,0.266]$. I leave out the technical details here, but I'm following the general meta-analysis methods for such setups, in Schweder and Hjort (2016, Ch. 13). One ingredient here is to model the events, from 2008 to 2017 and beyond, not to have the exactly same unfairness parameter value, as these may vary, slightly, from event to event; thus these are seen as $d_j\sim{\rm N}(d_0,\tau^2)$. A separate confidence distribution analysis of the spread parameter $\tau$ gives a 95% confidence interval $[0.023,0.136]$, with point estimate $\widehat\tau=0.069$.

To sum up, translating from statistics to speedskating, these analyses suggest the following.

• (i) Each World Sprint event has its own unfairness parameter, say $d$, which may vary from occasion to occasion, inside the band from a slim 0.058 seconds (almost fair) to 0.316 seconds (very imbalanced).
• (ii) The overall unfairness estimate is 0.187 seconds. It is highly significant, and the associated 99% confidence interval is very clearly to the right of zero, hence favouring the inner guy.
• (iii) The world becomes more fair (and a better one) when skaters are turning in two 1000-m races, one with start in inner and one with start in outer (and with the sum of the two race times determining the final result list). The start list for the 2nd race would then be the 1st race result list turned upside down, with a natural crescendo towards the best skaters near the end, and with each new pair, and the public, knowing precisely what is required to lead in the point-sum. This would be similar to what is in place for alpine skiing, for skijumping, and yet other sports. This kind of arrangement is already in place for the annual World Sprint Championships, but not for the Olympics, and not in the annual World Single Distances Championships. 

Notes & Concluding Remarks

Readers of my papers, over the years, whether about methodology or applications, have perhaps grown accustomed to a final section where I insist upon giving some "concluding remarks, some of which point to further research" (well, in cases where the journal editors have granted me this opportunity). Speedskating stories should not be exempt from this habit, so here I go.

A. What is it about speedskating that could bring an entire people together around the radio or television, women and men, young and old, rich and poor? What was it that compelled children to scribble down skate times and collect them in books? Why are the names of Kay Stenshjemmet, Jan Egil Storholt, Sten Stensen and Amund Sjøbrend etched in my memory 40 years after they hung up their skates for good, whereas I have long since forgotten the names of prominent politicians of the day or the teachers I had at school? – These thoughts are admittedly penned by Knausgård (February 2018), but they echo my own, and those of long generations of Norwegians. "It is when this struggle becomes plain that speedskating goes from sport to drama. Abruptly, the skater’s drawn-out inner battle becomes visibly external, will against body, body against clock, played out before thousands of eyes, transforming the final throes of the struggle against the body into a struggle against humiliation, against no longer remaining together, no longer being elegant, no longer being able to skate." Knausgård explains, to a few million culturally challenged Americans and others, that the point is not to win, but to struggle, to win with suffering (whether over yourself or your competitors).

These puritan, solitary, half-invisible aspects of speedskating are also made clearer in a glorious twelve-page photo essay about Redningsskøytene (also from February 2018), in Dagens Næringsliv's D2 Magazine. These dimensions are certainly present, when one hopes to explain or understand why the mysterious and partly introverted world of speedskating is so prominently at work, even on the pages of the nation's most serious novelists. I'd like to point out one more of these dimensions, though, regarding the Fields of Attraction: Statistics. Perhaps North Americans eat baseball statistics and batting averages for breakfast. A couple of generations of Norwegians learned numbers and calculus and statistics from speedskating. How should a proper 1500 m be set up, with which three laptimes following the 300-m opener, for a long-distance guy to optimise his chances of getting a bronze in the weighted-pointsum competition, given that he won the silver on the 5k and that his main opponent for said bronze, the 1500-m specialist, just did 1:46? 28000 Bislettians would quickly have done the maths and the stats and the predictions. Svein Sjøberg, professor of physics and of didactical learning for the sciences, who often laments the state of the nation when it comes to basic knowledge of maths and sciences (and who skated against Fred Anton Maier in the 7.28.1 March 1965 race, and against Jonny Nilsson in the 4.27.6 March 1963 race, as we all remember), says said nation needs to learn more of the basics, from, well, speedskating.  

B. Essentially the same 2 x 1000 m story has been written up as part of the Application Chapter 14 in Schweder and Hjort's 2016 CLP book (with sprint data up to 2014, whereas this blog post has been using such data also from seasons 2015, 2016, 2017), with some more details regarding the mathematics of the confidence distributions and configrams. I've also communicated the essential Olympic Unfairness findings to the ISU.

C. When I wrote up my Olympics-Changing 1994 report, the net wasn't fully invented yet, and I needed hard copies of all ISU result protocols to painstakingly put all inner-outer and lap time information into my files. Such data-gathering and file-organisational efforts are often enough part of the statistician's work tasks, also now. In 2018 it's relatively easy for me to find the relevant information, from ISU Results websites, but I still need an extra 25 minutes per World Sprint event to transform and translate and post-edit just the right information in just the right format (unless I devise a better net-scraping algorithm, which I haven't attempted), followed by careful outlier monitoring.

D. Given my bigaussian fixed-and-random effects model above, I can bypass some of the log-likelihood function complexities and work with a simpler regression model for the differences $D_i=y_{i,2}-y_{i,1}$ in terms of laptimes and the inner-outer covariate. This leads to a competing estimator, say $\widehat d_{\rm simple}$, which is typically just slightly less precise than my preferred $\widehat d_{\rm bigmodel}$ used above. The results coming out of such procedures are largely similar to those exposited above. I do prefer the full bivariate model, however, also since it provides other relevant information for the eager observer of speedskating, like the skater-to-skater and the inter-skater day-to-day variability, the effect of preliminary passing times on the final results, etc. In Hjort, Dahl, Steinbakk (2006), apropos, we spend quite a bit of time studying the intricate correlation structure for sets of 500-m and 1000-m results.

E. The analyses given here have neutrally but effectively pointed to and assessed the unfairness, without necessarily giving precise "but why?" explanations for the observed inner-advantage. Plausible reasons include those briefly mentioned, (i) the inner guy has a longer straight-ahead stretch to build speed at the start; (ii) the sprinters who use all their sprinting forces to have maximum speed for 900 m and then hope for the best for the remaining 100 m have clear difficulties with the last outer lane, when fatigue sets in and your muscles are burning and your body is close to the Knausgårdean collapse. There is a third reason favouring last inner, however, which I haven't attempted to analyse here, having to do with air resistance and the eating up your pairmate phenomenon on the last exchange stretch. Erben Wennemars and others make the point that if the two skaters are pretty close with one lap to go, then the last inner guy will win – actually with an average time advantage of 0.67 seconds, for that last lap alone (they appear to claim). Famously, you can't really plan to have luck with your partner in life, as it depends on how the partner behaves in the course of the life I mean race. But it's one more good reason for demanding 2 x 1000 m, with one start in inner and one in outer.

F. The speedskating world has changed in several ways over the past 20-25 years (some would say far too much). In addition to the traditional distances 500-m, 1000-m, 1500-m, 5k, 10k for men (and 500-m, 1000-m, 1500-m, 3k, 5k for ladies), there is now the team race (three skaters on a team, over eight laps, only inners, pursuing the team on the other side of the rink), mass start, and even three-leg-catapult-sprint. This means more events inside a crowded Olympic or World Championships time window, which also leads to a pressure of having fewer races. Thus the 2 x 500 m setup has been left, against the protest of many skaters, for the Olympics and also for the World Single Distances Championships; the 2 x 500-m plus 2 x 1000-m setup of things, is still being kept for the World Sprints, however. The implication is that even if skaters and specialists agree with me (and most do), it'll be hard to squeeze in another 1000-m for the Olympics.

G. Several Olympic and World Championships 1000-m and 500-m winners have published books about their lives and careers (or have had journalists writing about them), among them Dan Jansen, Catriona LeMay Doan (whose honour I once had to defend, in Aftenposten, after that newspaper's sexist nakendamehartkornslåingoppslag about her – "Det er her jeg må trekke mitt slitte riddersverd, i erkjennelse av at landets militante feminister sannsynligvis har måttet gi opp sin interesse for hurtigløp på skøyter etter den stemoderlige behandling denne idrett nå har i media. Avsnittet over er nemlig grovt feilaktig og krenkende."), Yevgeny Grishin, Erhard Keller, Peter Mueller, Annie Friesinger, Eric Heiden. They all make comments about the inherent dangers of the outer lane in the 1k. Dianne Holum (1984, p. 225), for example, rather clearly blames starting in the outer on her Sunday-1000-m for losing the 1972 World Championships to Monika Pflug.

H. The model I've built and used for these analyses is moderately complicated by having fixed and random effects, and I've worked out estimation and assessment methods and algorithms from scratch, so so speak. The model is however also a special case of the so-called linear mixed models, for which methodology and R packages have been worked out, from general perspectives. See also the FIC for Whales work, where Céline Cunen, Lars Walløe and I hammer out new methods and formulae for model selection and model assessment, for these classes of models, and where the FIC machinery we develop may be applied also for the World Sprint Championships data.

Thanks

I am grateful to all the participants of these ten years of annual World Sprint Bigaußian Fixed and Random Effects Championships, for their spellbinding & mindboggling efforts, which continue to be a joy to behold. I also thank Céline Cunen and the others at the FocuStat research group for discussions of several of the pertinent methodological points, and also the multitude of eager contributors of knowledge and tireless discussants of all historical matters, small and big, in the vibrant and broadminded Forum for Skøytehistorie on Facebook.

References

Bjørnsen, K. 13 år med Kuppern & Co. Nasjonalforlaget, Oslo.

Cunen, C., Walløe, L. and Hjort, N.L. (2018). Focused model selection for linear mixed models, with an application to whale ecology. (Submitted for publication, February 2018.)

Friesinger, A. Mein Leben, mein Sport, meine besten Fitness-Tipps. Goldmann, Berlin.

Гришин, Е.Р. (1969). 500 метров. Publisher: Молодая гвардия.

Gundersen, J.-A.Ø. (2018). Redningsskøytene. 12-page report and essay on the new wave of Norwegian top skaters, in Dagens Nærlingslivs D2 magasin.

Heiden, E. (1979). For moro skyld. Cappelen, Oslo.

Hjort, N.L. (1994). Should the Olympic speed skaters run the 500 m twice? Statistical Research Report, Department of Mathematics, University of Oslo.

Hjort, N.L., Dahl, F.A. and Steinbakk, G.H. (2006). Post-processing posterior predictive p-values. Journal of the American Statistical Association 101, 1157-1174.

Holum, D. (1984). The Complete Handbook of Speed Skating. High Peaks Cyclery, Lake Placid.

ISU Results (International Skating Union). Results from international championships, in particular from the World Sprint Championships, can be found here, for the 2017 event used above, and here, for other seasons (soon to include the 2018 Olympics and the March 2018 Changchun World Sprint).

Jansen, D. (1994). Full Circle. Villard Books, New York.

Keller, E. (1968). 74 Schritte zum Ziel. Copress-Verlag München.

Knausgård, K.O. (2018). The Hidden Drama of Speedskating. Essay, The New York Times, 1-Feb-2018.

LeMay Doan, C. (2002). Going for Gold. McClelland & Stewart, Toronto.

Rosa, D. and Hjort, N.L. (1999). Who Won? The Annual Speedskating Race of the Burg of Ducks. Speedskating World. (Also included in Don Rosa Collected Works, 2011.)

Scholten, M. (2005). Op dun ijs - Het verhaal van Peter Mueller. Arko Sports Media Bv, Amsterdam.

Schweder, T. and Hjort, N.L. (2016). Confidence, Likelihood, Probability: Statistical Inference With Confidence Distributions. Cambridge University Press, Cambridge.

Wennemars, E. (2017). Wennemars legt uit: het geheim van de binnenboch. The multiple World Sprint Champion gives a pedagogical tv lecture (which for educational reasons, I suppose, lasts precisely as long as Grishin's 1955 world record time for the 1k) to the Dutch about The Secret of the inner lane.